E.T.C.
On this page I introduce the simulation tools I have built so far. Since there is no uniformity in the genres, I have grouped them all together under "etc." :-). I've been updating quite frequently recently. I'm not sure how long I can keep up these updates, but I'd like to keep trying as long as I'm still interested. The tools I've introduced are as follows:
Lens Meter Simulation tool
Differential Equations drawing tools
Rendering Surfaces with Hidden Line Meshes
* These tools are introduced in order of update.
10/13/25

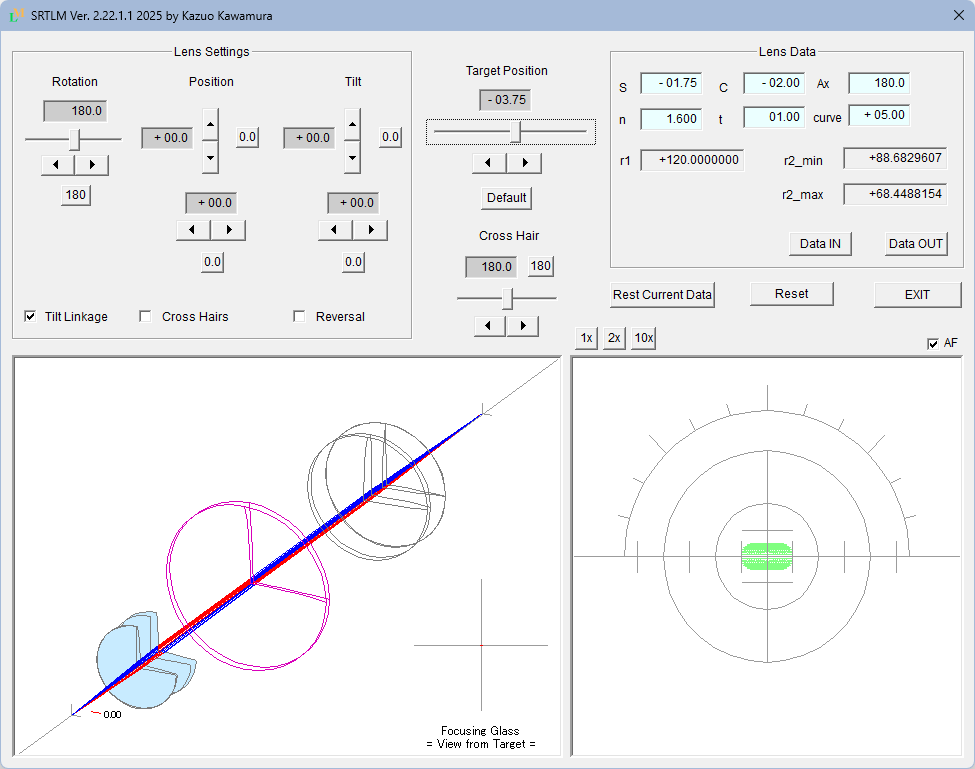
The source code for the lens meter simulation tool "SRTLM" has been released.
You can get it from GitHub by clicking "Lensmeter-Simulation-Tool" below.
For executable files and documentation, please refer to SRTLM_En as before.
[E.T.C.] - SRTLM_En
09/10/25

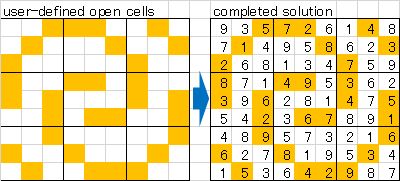
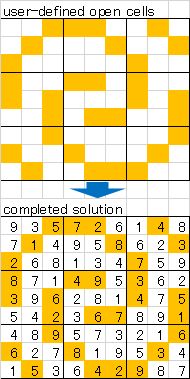
I have created an another version of the Sudoku puzzle generator tool using an Excel macro.
This another method allows you to create puzzles with freely defined patterns. And generate the completed form.
This another method allows you to create puzzles with freely defined patterns. And generate the completed form.
This tool can be downloaded as freeware.
[E.T.C.] - SudokuAnother Method
09/10/25

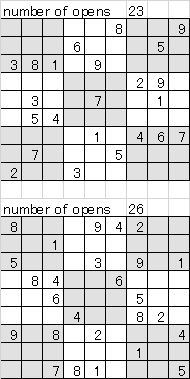
Updated the Sudoku puzzle generation tool: Added the ability to save generated puzzles.
This tool can be downloaded as freeware.
[E.T.C.] - Sudoku Update01
09/10/25

I have created a tool for generating Sudoku puzzles using an Excel macro.
This tool can be downloaded as freeware.
[E.T.C.] - Sudoku
03/26/25

For this site, this is a rather unique topic.
I seriously considered
"the mystery of the seafarers who transported obsidian by boat
from Kozushima Island in the Izu Islands to the mainland 38,000 years ago. "
[E.T.C.] - Voyagers of 38,000 years ago
09/08/24

I have released a page that plots graphs (surfaces) of functions of two variables
using a mesh with hidden line.
Select a function on the select box to plot the surface.
Below is link to explanatory page:
The following link directly jumps to the drawing page:
Select an function from the dropdown box and click [Start] to begin plot the surface.
[E.T.C.] - Plotting Surfaces Using Hidden Line Meshes
Select an function from the dropdown box and click [Start] to begin plot the surface.
[E.T.C.] - Plotting Surfaces Using Hidden Line Meshes
In addition to the pre-prepared functions,
the plotting page also has a feature that allows users to enter function directly and render graphs.
By entering the function you want to check, you can quickly check the shape of the surface.
07/06/24

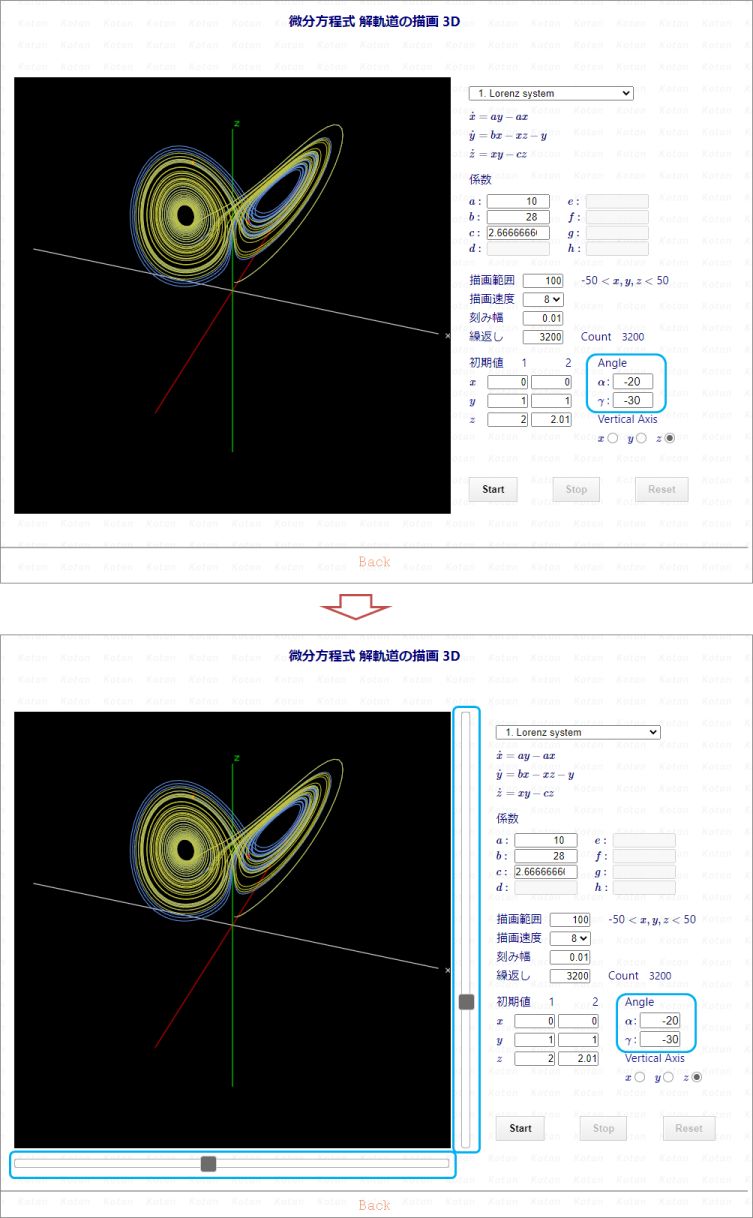
On the page for drawing the solution trajectory of a differential equation (3D),
I have changed the operation using the spin button to a slider bar.
Previously, the coordinate system could be rotated after drawing using the spin button.
However, this was difficult to use, so we have modified it
so that it can be rotated smoothly using the slider.
06/24/24

The following features have been added to the page for drawing solution trajectories of differential equations (2-dimensional).
Previously, the drawing page only allowed you to draw solution orbits for pre-set equations,
but by selecting "User Input", you can freely set equations.
You can quickly investigate the solution orbits, equilibrium points, etc.
of the equations you want to check.
This is a feature I personally wanted on the drawing page, so I hope you will find it useful.
Refer to User defined Equations for details.
05/11/24

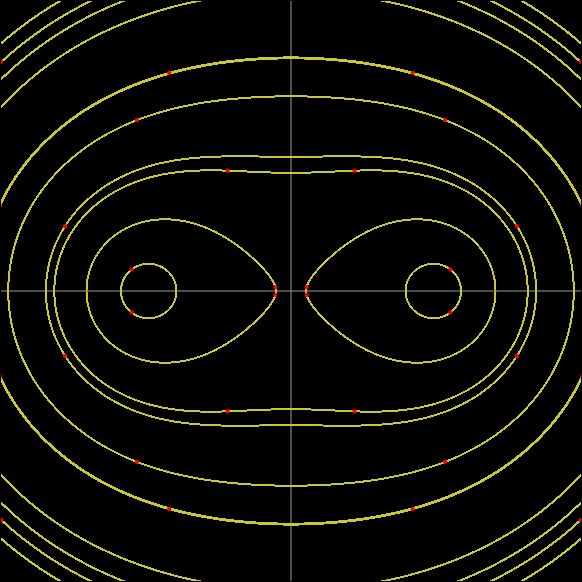
The following equation has been added to the page for drawing solution trajectories of differential equations (2-dimensional).
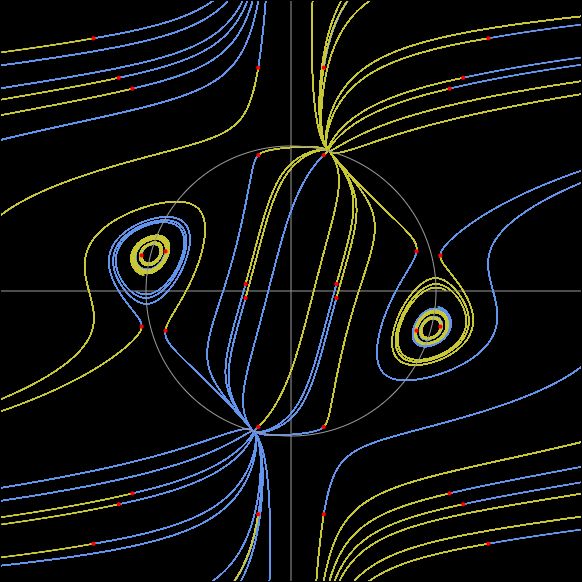
[04] 2-Dimensional Autonomous System Examples
- Hamilton system 1 : Cassini oval
- Hamilton system 2 : folium of Descartes
- Hamilton system 3 : derivatives of "folium of Descartes"
- Hamilton system 4 : Case of the periodicity of trigonometric functions is reflected
04/28/24

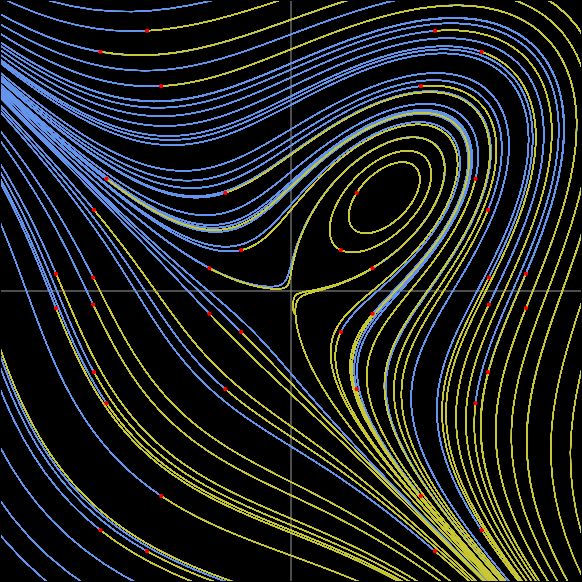
The following equation has been added to the page for drawing solution trajectories of differential equations (2-dimensional).
[04] 2-Dimensional Autonomous System Examples
-
Limit Cycle: example 4 Linear approximation with purely imaginary numbers - 1
Case of no limit circle exists -
Limit Cycle: example 5 Linear approximation with purely imaginary numbers - 2
case : an infinite number of limit circles appear
04/15/24

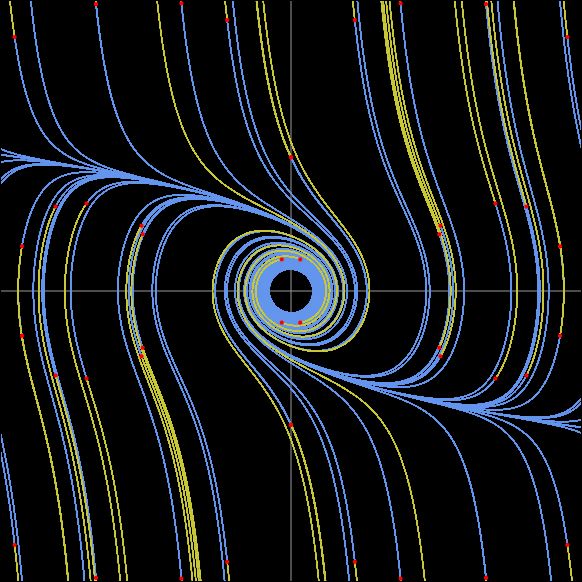
The following equation has been added to the page for drawing solution trajectories of differential equations (2-dimensional).
[04] 2-Dimensional Autonomous System Examples
- Limit Cycle Example 1
- Limit Cycle Example 2: von del Pol
- Limit Cycle Example 3: Lemniscate
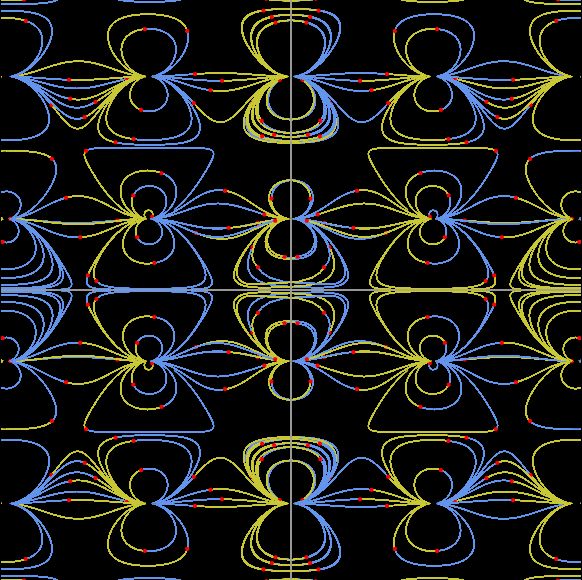
03/23/24

The following equation has been added to the page for drawing solution trajectories of differential equations (2-dimensional).
[04] 2-Dimensional Autonomous System Examples
- Autonomous system with periodic equilibrium points on x-axis
- Autonomous system with 8 equilibrium points on the circumference
- Autonomous system with 8 equilibrium points on a plane
03/10/24

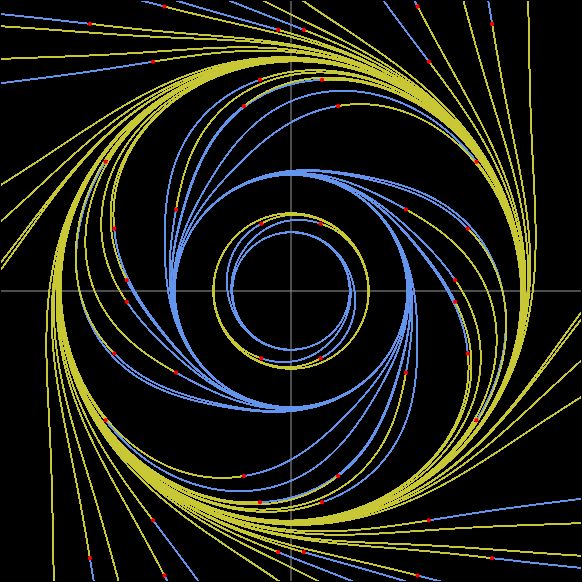
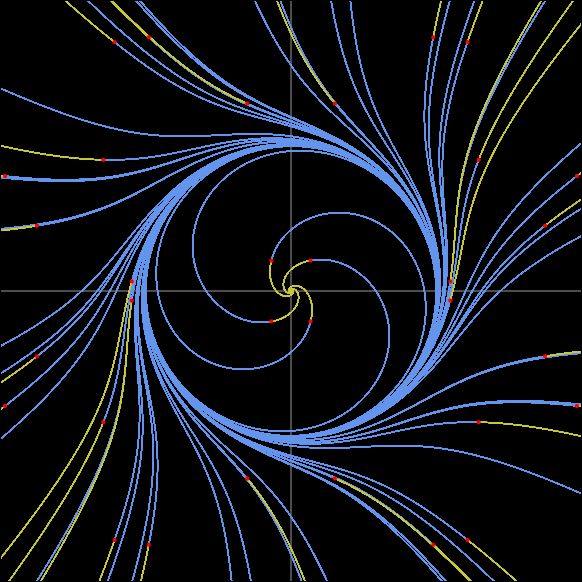
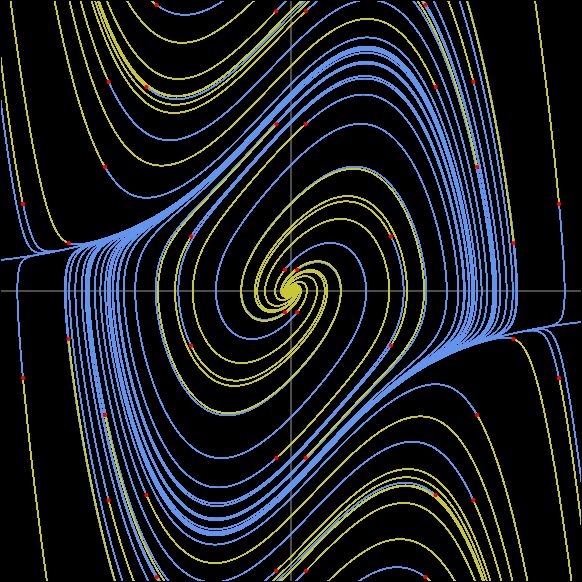
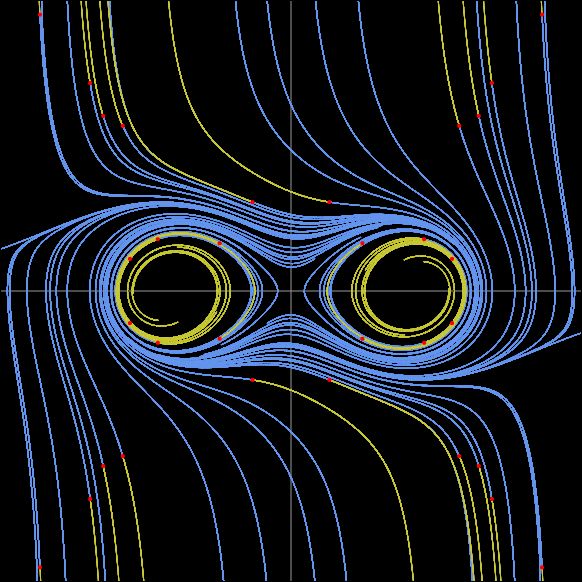
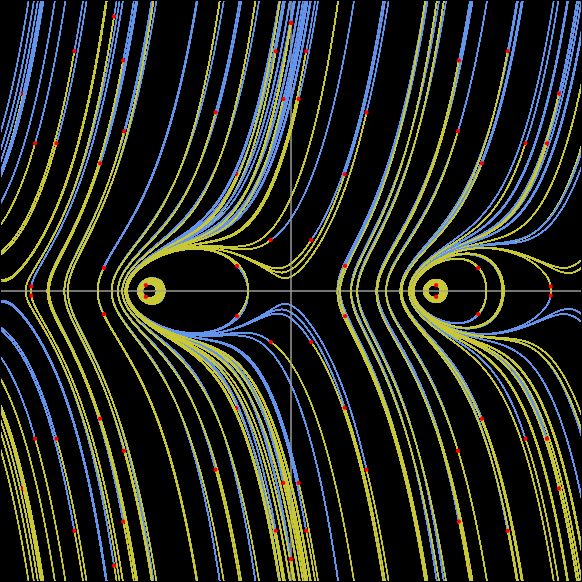
I have published a page for drawing solution trajectories of (2-dimensional) differential equations.
When you select an equation on the page, it dynamically plots the solution trajectory.
Originally, I had built a drawing tool in C#, but I wanted to directly draw on the web, so I rewrote it in JavaScript. However, I only had minimal experience with JavaScript about 10 years ago, and I encountered several "Hmm, how do I do this again?" moments.
So, I utilized ChatGPT. I want to explicitly mention here that using ChatGPT significantly saved me time in looking up things while coding.
Originally, I had built a drawing tool in C#, but I wanted to directly draw on the web, so I rewrote it in JavaScript. However, I only had minimal experience with JavaScript about 10 years ago, and I encountered several "Hmm, how do I do this again?" moments.
So, I utilized ChatGPT. I want to explicitly mention here that using ChatGPT significantly saved me time in looking up things while coding.
The equations featured on this page are based on excerpts from
"Differential Equations on Personal Computers" by Mitsuo Morimoto.
Although Morimoto has introduced many equations, I haven't completed confirmation on my end yet.
Once confirmed, I intend to gradually add them.
Below is link to explanatory page:
Clicking "To Plot" in the explanation section of each equation will transition you to the drawing page with the necessary data set for plotting. Clicking the [START] button begins plotting the solution curves.
Clicking "To Plot" in the explanation section of each equation will transition you to the drawing page with the necessary data set for plotting. Clicking the [START] button begins plotting the solution curves.
The following link directly jumps to the drawing page:
Select an equation from the dropdown box and click [Start] to begin plottinf the solution trajectory.
Select an equation from the dropdown box and click [Start] to begin plottinf the solution trajectory.
On the drawing page, you can configure the following settings:
- Coefficients (modifiable parameters vary for each equation)
- Plotting range
- Increment width (width of elapsed time)
- Iteration count (number of executions of the increment width)
- Approximation method (Euler's method, Modified Euler's method, 4th Order Runge-Kutta method)
03/10/24

I have also published a page for plotting solution trajectories of differential equations with 3D.
It operates similarly to the 2D version of the differential equation page,
and I would like to note that I utilized ChatGPT in the same manner.
The equations showcased on this page primarily focus on chaotic trajectories.
If I find interesting equations, I plan to increase the samples.
Below are links to explanatory pages:
Clicking "To Plot" in the explanation section of each equation
will transition you to the drawing page with the necessary data set for plotting.
Clicking the [START] button begins plotting the solution curves.
After drawing, you can observe the solution trajectories from various angles by changing the Angle and Vertical Axis.
The following link directly jumps to the drawing page:
Select an equation from the dropdown box and click [Start] to begin drawing the solution trajectory. After drawing, you can observe the solution trajectories from various angles by changing the "Angle" and "Vertical Axis".
Select an equation from the dropdown box and click [Start] to begin drawing the solution trajectory. After drawing, you can observe the solution trajectories from various angles by changing the "Angle" and "Vertical Axis".
On the drawing page, you can configure the following settings:
- Coefficients (modifiable parameters vary for each equation)
- Plotting range
- Increment width (width of elapsed time)
- Iteration count (number of executions of the increment width)
- Initial values (can specify 2 points)
- Angle (left-right, up-down)
- Vertical Axis (default is z-axis, but you can also choose x-axis and y-axis)
08/01/23

Lens meter simulation tool "SRTLM" has been released.
This tool can be downloaded as freeware.
[E.T.C.] - SRTLM_En